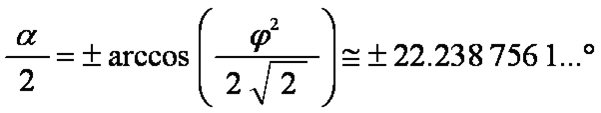The "Jitterbug"
Rotation axes of the triangles are the 4 vertex-to-opposite-vertex axes of the Cube.
Two motions: 1) All triangles rotate, 2) Polar opposite triangles don't rotate.
Jitterbug vertices trace out portions of ellipses.
Ellipse = Slice of a Cylinder
The vertices are on the surfaces of 4 intersecting cylinders.
Portion of the ellipse and the polyhedron positions.
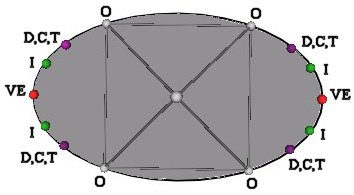
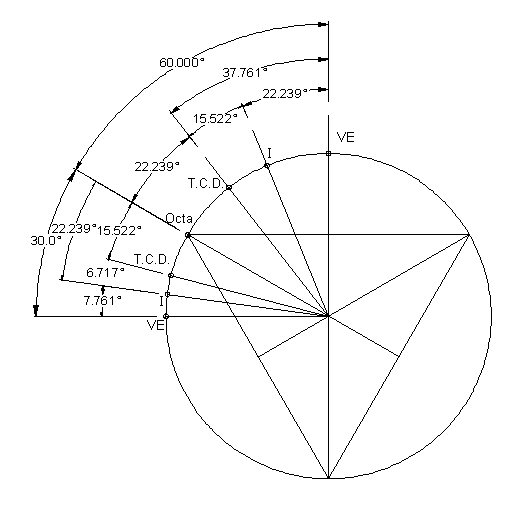
By how much is the Jitterbug's triangles rotated for the various polyhedra positions?
VE to Icosahedron
Octahedron to Dodecahedron position
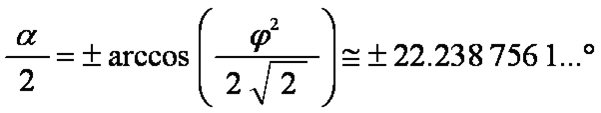
VE to Dodecahedron
Octahedron to Icosahedron position

If the vertices are allowed to move completely around the ellipse
another set of the polyhedra positions are found.

Summary of Angles
Copyright September, 2007 by Robert W. Gray