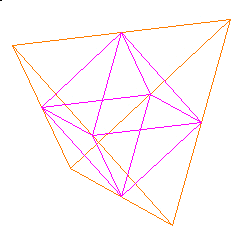
We have seen how the pattern can be constructed from a tetrahedron. We divided the tetrahedron's edges in half which defined an octahedron inside the tetrahedron.

We then drew circles on each of the 4 tetrahedron's faces.
Instead of doing that, we will vibrate the octahedron's edges.

(I have increased the arc radius from that of the construction using circles so that the vibrations will not intersect each other.)
This gives us


We see that the edges of the tetrahedron are not involved at all so we delete them.

When we trimed the 4 circles in the previous tetrahedron construction, we had to do it in a special way. We find that all the trimmed parts belonged to the equator of the octahedron. We can change the color of the, now vibrating, octahedron equator to light blue.


The remainning octahedron edges, in green, is the "pattern" with its "edges" vibrating in 3-space. (The red curve in the next illustration attempts to highlight the patern path.)

Lynnclaire told me that when the pattern is formed, the "energy" bounces around in a 12 90-degree path. I thought that this might involve the octahedron since the octahedron can be formed by interesting 3 squares. Each square has 4 90-degree angles, so there would be a total of 12 90-degree angles invovled in the octahedron. (Actually, there are more than 12 90-degree angles involved in the octahedron since the central angles are also 90-degree angles. And there are 12 central angles.)
This may help to explain the "breathing" motion Lynnclaire describes with the pattern. If each "piece" of the pattern is a bit of vibrating "stuff", and there are 8 such bits, then in its most stable configuration would form an octahedron. Four more bits of vibrating stuff would be needed at the octahedron's equator to further stablize the structure.
Note that from Mathermatical Physics we find that when you vibrate the surface of a sphere, a spherical octahedron can emerge. This is one of the spherical harmonics.
The knot aspect of all this has not been addressed here. The only thing I need to point out is that the 2 polar vertices of the octahedron where the loops of the pattern seem to merge into a single point would actually weave around each other. Like a tensegrity structure, the vertices do not merge into a single point. This allows for all of the above to be considered as knotted.
And this allows the above structures to move in the "breathing" motion.
Usage Note: My work is copyrighted. You may use my work but you may not include my work, or parts of it, in any for-profit project without my consent.