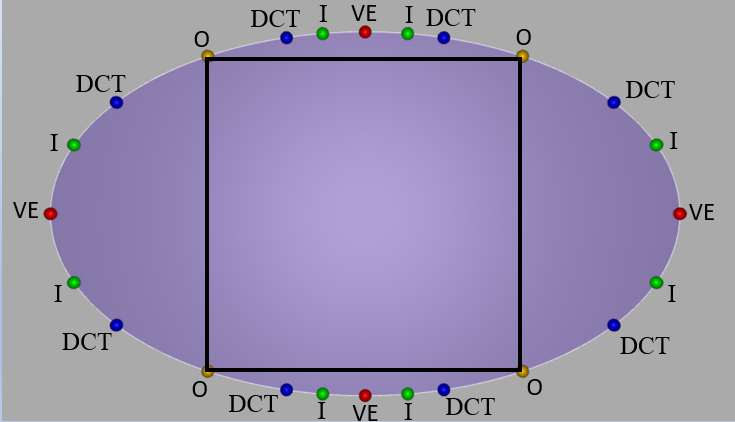
Here is an interactive web page that lets you explore the Jitterbug and some of the associated polyhedra.
The Jitterbug has "special" positions that are associated with various polyhedra. Specifically, the Tetrahedron, Cube, Octahedron, Dodecahedron, Icosahedron and the VE (Cuboctahedron).
There are "outer" and "inner" versions of these polyhedra, depending on the position of the Jitterbug's vertices around the ellipses that map out the vertex's motion.

The volume equations will be written in terms of the Octahedron's (Jitterbug's) edge length.
The equation for the volume of the initial Octahedron is $$ Vol_{O} = \dfrac{\sqrt{2}}{3} EL_{O}^{3} $$

The equation for the volume of the outer Dodecahedron is $$ Vol_{D} = \dfrac{5+\sqrt{5}}{2} EL_{O}^{3} $$
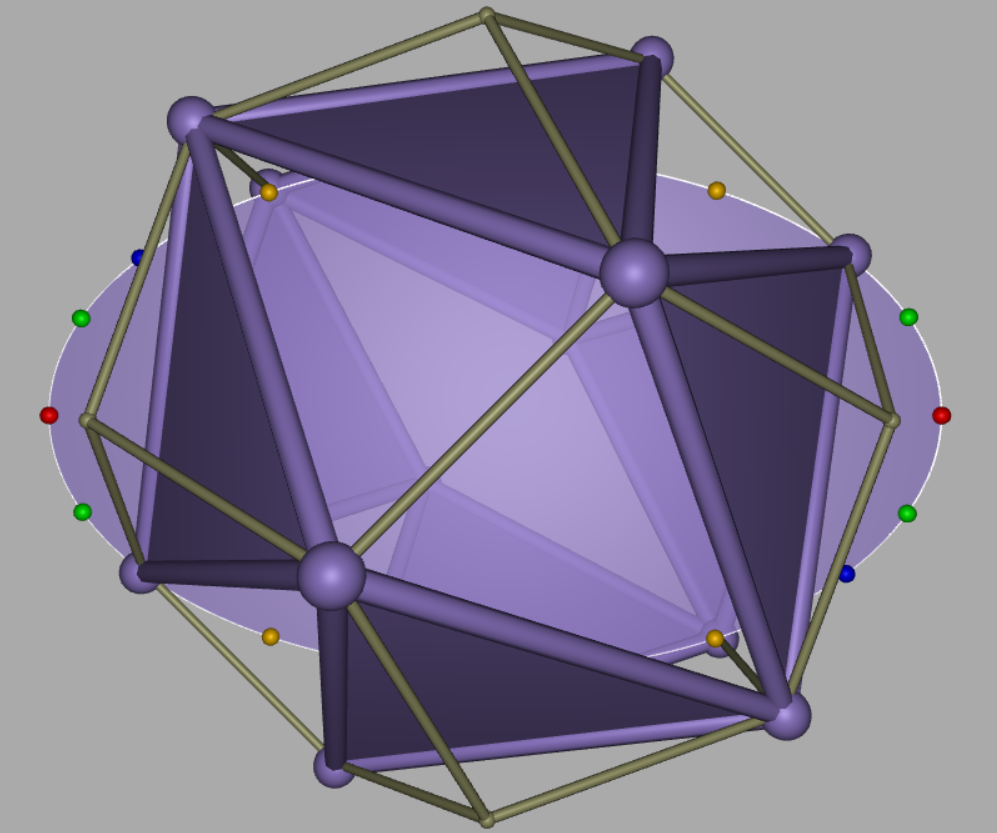
The equation for the volume of the outer Cube is $$ Vol_{C} = EL_{O}^{3} $$

The equation for the volume of the outer Tetrahedron is $$ Vol_{T} = \dfrac{1}{3} EL_{O}^{3} $$
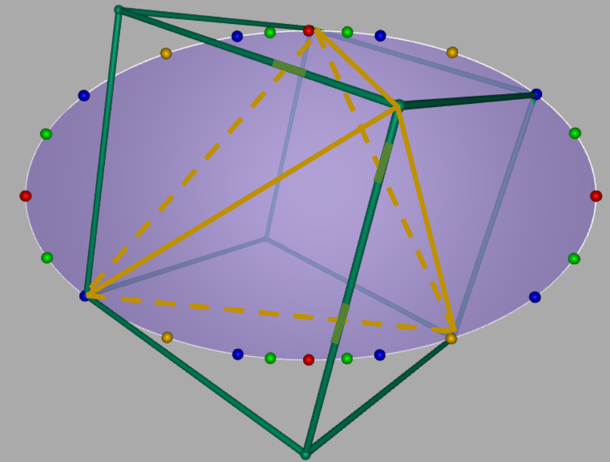
The equation for the volume of the outer Icosahedron is $$ Vol_{I} = \dfrac{5 (3+\sqrt{5})}{12} EL_{O}^{3} $$

The equation for the volume of the outer VE (Cuboctahedron) is $$ Vol_{VE} = \dfrac{5 \sqrt{2}}{3} EL_{O}^{3} $$
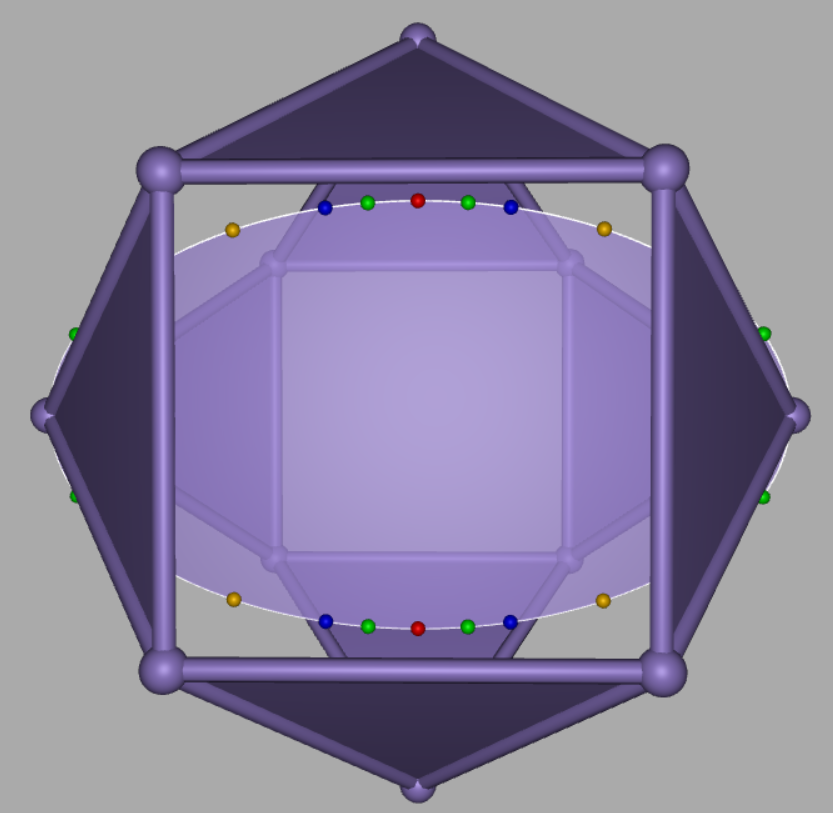
We now provide the volume equations for the "inner" polyhedra. Again, these will be provided in terms of the Jitterbug's (Octahedron's) edge length. The edge lengths of the polyhedra in terms of the Octahedron's edge length is also given.
The edge length of the inner Dodecahedron in terms of the Jitterbug's (Octahedron's) edge length is $$ EL_{D} = \dfrac{\sqrt{3-\sqrt{5}}}{2} EL_{O} \approx 0.437016 EL_{O} $$
The equation for the volume of the inner Dodecahedron is $$ Vol_{D} = \dfrac{15+7\sqrt{5}}{\sqrt{2}(1+\sqrt{5})^3} EL_{O}^{3} \approx 0.639584 EL_{O}^{3} $$
The edge length of the Cube in the Dodecahedron is given by $$ EL_{C} = \dfrac{1+\sqrt{5}}{2} EL_{D} $$ The edge length of the Dodecahedron in terms of the Jitterbug (Octahedron) edge length is given above as $$ EL_{D} = \dfrac{\sqrt{3-\sqrt{5}}}{2} EL_{O} $$ So $$ EL_{C} = \dfrac{1+\sqrt{5}}{2} \dfrac{\sqrt{3-\sqrt{5}}}{2} EL_{O} $$
The equation for the volume of the inner Cube is $$ Vol_{C} = \dfrac{1}{8}(1+\sqrt{5})\left(\sqrt{3-\sqrt{5}}\right) EL_{O}^{3} $$

The equation for the volume of the inner Tetrahedron is $$ Vol_{T} = \dfrac{1}{24}(1+\sqrt{5})\left(\sqrt{3-\sqrt{5}}\right) EL_{O}^{3} $$

The edge length of the inner Icosahedron in terms of the Jitterbug's (Octahedron's) edge length is $$ EL_{I} = \dfrac{\sqrt{2}}{\sqrt{3+\sqrt{5}}} EL_{O} $$
The equation for the volume of the inner Icosahedron is $$ Vol_{I} = \dfrac{5}{3\sqrt{2(3+\sqrt{5})}} EL_{O}^{3} $$

The edge length of the inner VE (Cuboctahedron) in terms of the Jitterbug's (Octahedron's) edge length is $$ EL_{VE} = \dfrac{1}{\sqrt{3}} EL_{O} $$
The equation for the volume of the inner VE (Cuboctahedron) is $$ Vol_{VE} = \dfrac{5 \sqrt{2}}{9 \sqrt{3}} EL_{O}^{3} $$

Usage Note: My work is copyrighted. You may use my work but you may not include my work, or parts of it, in any for-profit project without my consent.