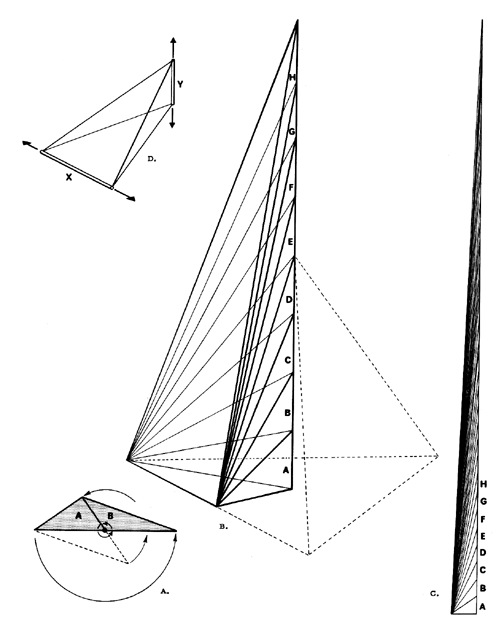
Fig. 923.10 Constant Volume of A and B Quanta Modules:
- A comparison of the end views of the A and B Quanta
Modules shows that they have equal volumes
by virtue of the fact that they have equal base areas
and identical altitudes.
- It follows from this that if a line, originating
at the center of area of the triangular base of a regular
tetrahedron, is projected through the apex of the tetrahedron
to infinity, is subdivided into equal
Increments, it will give rise to additional Modules
to infinity. Each additional Module will have the
same volume as the original A or B Module, and as the
incremental line approaches infinity the
Modules will tend to become lines, but lines still having
the same volume as the original A or B
Module
- End view shows Modules beyond the H Module shown
in (B).
- The two discrete members X and Y can move anywhere
along their respective axes and the volume
of the irregular tetrahedron remains constant. The other
four edges vary as required.
|