 Energy-valve Functioning of Outer Shell of Nuclear
Domains
Energy-valve Functioning of Outer Shell of Nuclear
Domains
466.00  Energy-valve Functioning of Outer Shell of Nuclear
Domains
Energy-valve Functioning of Outer Shell of Nuclear
Domains
 Fig. 466.00  Fig. 466.01 |
466.01
 An earlier version of Fig. 466.01 was first published
by the author in 1944: it
illustrates the energy-valving aspects of the closest-packed
spheres interfunctionings as
they occur within the three-frequency, 92-ball outer
layer of the vector equilibrium as it
"jitterbuggingly" skew-transforms into the icosahedral
state, then returns to the vector
equilibrium state, passes through, and again transforms
to the alternately skewed
icosahedral state__ repeat and repeat.
An earlier version of Fig. 466.01 was first published
by the author in 1944: it
illustrates the energy-valving aspects of the closest-packed
spheres interfunctionings as
they occur within the three-frequency, 92-ball outer
layer of the vector equilibrium as it
"jitterbuggingly" skew-transforms into the icosahedral
state, then returns to the vector
equilibrium state, passes through, and again transforms
to the alternately skewed
icosahedral state__ repeat and repeat.
|

|
466.02
 The 90-degree interalignment of the 16 balls of any
one of the six square
faces of the vector equilibrium (Fig. B) is inherently
unstable. The 16 balls resolve their
instability by forming any one of two alternate types
of most closely packed diamonds
(Figs. D and E) with either a short cross axis or a
long diagonal axis. Both types are
equiedged, equiarea, and most densely packed, and they
occupy less area than their
equiedged square counterparts. This is quickly evidenced
geometrically because both the
square (Fig. B) and the diamond (Fig. D) have the same-length
base edge XY, but the
altitude WZ of the square is greater than the altitude
Z of the diamond.
The 90-degree interalignment of the 16 balls of any
one of the six square
faces of the vector equilibrium (Fig. B) is inherently
unstable. The 16 balls resolve their
instability by forming any one of two alternate types
of most closely packed diamonds
(Figs. D and E) with either a short cross axis or a
long diagonal axis. Both types are
equiedged, equiarea, and most densely packed, and they
occupy less area than their
equiedged square counterparts. This is quickly evidenced
geometrically because both the
square (Fig. B) and the diamond (Fig. D) have the same-length
base edge XY, but the
altitude WZ of the square is greater than the altitude
Z of the diamond.
|

|
466.03
 As displayed in a planar array, Fig. A, there is an
apex sphere K
surroundingly shared by the innermost corners (vertexes)
of two square-faced, 16-ball
grids, M and N, as well as by the two diamonds—the short-axis
diamond E and the long-
axis diamond D.
As displayed in a planar array, Fig. A, there is an
apex sphere K
surroundingly shared by the innermost corners (vertexes)
of two square-faced, 16-ball
grids, M and N, as well as by the two diamonds—the short-axis
diamond E and the long-
axis diamond D.
|

|
466.04
 The apex sphere K's neighboring spheres are uncomfortable
because K is
surrounded by seven spheres and not six. Only six can
closest pack around one in any
given plane. One of the two adjacent spheres M or N
from the two square-faced grids will
get pushed in, and the other one will be pushed out,
depending upon which way the
vector-equilibrium-to-icosahedron jitterbug transformation
is rotating around apex sphere
K. The "in-and-out" pumping of spheres M and N acts
as an energy-propagating valve.
The apex sphere K's neighboring spheres are uncomfortable
because K is
surrounded by seven spheres and not six. Only six can
closest pack around one in any
given plane. One of the two adjacent spheres M or N
from the two square-faced grids will
get pushed in, and the other one will be pushed out,
depending upon which way the
vector-equilibrium-to-icosahedron jitterbug transformation
is rotating around apex sphere
K. The "in-and-out" pumping of spheres M and N acts
as an energy-propagating valve.
|

|
466.05
 Fig. C is a plan view of the closest-sphere-packing
manifestation of any one
of the vector equilibrium's four pairs of nuclear tetrahedra
as they commence to torque in
the jitterbug process. An isometric sketch of this net
39-ball aggregation is given at Fig.
466.31
Note that this torqued pair of nuclear tetrahedra
employs three of the vector
equilibrium's six axes. The two unengaged axes of the
equator are starved and
inoperative.
Fig. C is a plan view of the closest-sphere-packing
manifestation of any one
of the vector equilibrium's four pairs of nuclear tetrahedra
as they commence to torque in
the jitterbug process. An isometric sketch of this net
39-ball aggregation is given at Fig.
466.31
Note that this torqued pair of nuclear tetrahedra
employs three of the vector
equilibrium's six axes. The two unengaged axes of the
equator are starved and
inoperative.
|

|
466.10
 High-frequency Sphericity Approaches Flatness
High-frequency Sphericity Approaches Flatness
|

|
466.11
 Where we have six balls in a planar array closest
packed around one nucleus,
we produce six top and six bottom concave tetrahedral
valleys surrounding the nucleus
ball. We will call the top set of valleys the northern
set and the bottom set the southern
set. Despite there being six northern valleys we find
that we can nest only three close-
packed (triangulated) balls in the valleys. This is
because we find that the balls nesting on
top of the valleys occupy twice as much planar area
as that afforded by the six tetrahedral
valleys. Three balls can rest together on the top in
omni-close-packed tangency with one
another and with the seven balls below them; and three
balls can similarly rest
omniintertangentially in the bottom valleys as their
top and bottom points of tangency
bridge exactly across the unoccupied valleys, allowing
room for no other spheres. This
produces the symmetrical nuclear vector equilibrium
of 12 closest-packed spheres around
one. (See Fig. 466.13A.)
Where we have six balls in a planar array closest
packed around one nucleus,
we produce six top and six bottom concave tetrahedral
valleys surrounding the nucleus
ball. We will call the top set of valleys the northern
set and the bottom set the southern
set. Despite there being six northern valleys we find
that we can nest only three close-
packed (triangulated) balls in the valleys. This is
because we find that the balls nesting on
top of the valleys occupy twice as much planar area
as that afforded by the six tetrahedral
valleys. Three balls can rest together on the top in
omni-close-packed tangency with one
another and with the seven balls below them; and three
balls can similarly rest
omniintertangentially in the bottom valleys as their
top and bottom points of tangency
bridge exactly across the unoccupied valleys, allowing
room for no other spheres. This
produces the symmetrical nuclear vector equilibrium
of 12 closest-packed spheres around
one. (See Fig. 466.13A.)
|

|
466.12
 The three balls on the top can be lifted as a triangular
group and rotated 60
degrees in a plane parallel to the seven balls of the
hexagonal equatorial set below them;
this triangular group can be then set into the three
previously vacant and bridged-over
valleys. As this occurs, we have the same 12 spheres
closest packed around one with an
overall arrangement with the two triangular sets of
three on the top, three on the bottom,
and six around the equator. The top and the bottom triangular
sets act as poles of the
system, which__ as with all systems__ has inherent free
spinnability. In both of the two
alternate valley occupations the northern polar triangle
is surrounded alternately by three
squares and three triangles, reading alternately__ triangle,
square, triangle, square,
triangle, square.
(See Fig. 466.13B.)
The three balls on the top can be lifted as a triangular
group and rotated 60
degrees in a plane parallel to the seven balls of the
hexagonal equatorial set below them;
this triangular group can be then set into the three
previously vacant and bridged-over
valleys. As this occurs, we have the same 12 spheres
closest packed around one with an
overall arrangement with the two triangular sets of
three on the top, three on the bottom,
and six around the equator. The top and the bottom triangular
sets act as poles of the
system, which__ as with all systems__ has inherent free
spinnability. In both of the two
alternate valley occupations the northern polar triangle
is surrounded alternately by three
squares and three triangles, reading alternately__ triangle,
square, triangle, square,
triangle, square.
(See Fig. 466.13B.)
|
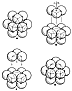 Fig. 466.13 |
466.13
 In one polar triangular valley occupation the squares
of the northern
hemisphere will be adjacent to the triangles of the
southern hemisphere. This is the vector-
equilibrium condition. In the alternate valley nesting
position at the equator the equatorial
edges of the squares of the northern hemisphere will
abut the squares of the southern
hemisphere, and the triangles of the northern hemisphere
will abut those of the southern,
producing a polarized symmetry condition. In the vector-equilibrium
condition we have
always and everywhere the triangle-and-square abutments,
which produces a four-
dimensional symmetry system.
(See Sec. 442
and Fig.
466.13C.)
In one polar triangular valley occupation the squares
of the northern
hemisphere will be adjacent to the triangles of the
southern hemisphere. This is the vector-
equilibrium condition. In the alternate valley nesting
position at the equator the equatorial
edges of the squares of the northern hemisphere will
abut the squares of the southern
hemisphere, and the triangles of the northern hemisphere
will abut those of the southern,
producing a polarized symmetry condition. In the vector-equilibrium
condition we have
always and everywhere the triangle-and-square abutments,
which produces a four-
dimensional symmetry system.
(See Sec. 442
and Fig.
466.13C.)
|

|
466.14
 There is then a duality of conditions of the same
12 nucleus-surrounding first
omni-inter-closest-packed layer: we have both a polarized
symmetry phase and an
equilibrious symmetry phase. Under these alternate conditions
we have one of those
opportunities of physical Universe to develop a pulsative
alternation of interpatterning
realizations, whereby the alternations in its equilibrium
phase do not activate energy, while
its polarized phase does activate energetic proclivities.
The equilibrious phase has no
associative proclivities, while the polarized phase
has associative proclivities. In the
polarized phase we have repulsion at one end and attraction
at the other: potential
switchings on and off of energetic physical Universe.
(See Figure 466.13D.)
There is then a duality of conditions of the same
12 nucleus-surrounding first
omni-inter-closest-packed layer: we have both a polarized
symmetry phase and an
equilibrious symmetry phase. Under these alternate conditions
we have one of those
opportunities of physical Universe to develop a pulsative
alternation of interpatterning
realizations, whereby the alternations in its equilibrium
phase do not activate energy, while
its polarized phase does activate energetic proclivities.
The equilibrious phase has no
associative proclivities, while the polarized phase
has associative proclivities. In the
polarized phase we have repulsion at one end and attraction
at the other: potential
switchings on and off of energetic physical Universe.
(See Figure 466.13D.)
|

|
466.15
 When modular frequency enters into the alternately
vector
equilibriumępolarized conformations, the vertexes of
the multifrequenced nuclear system
are occupied by uniradius spheres, whereat it is evidenced
that the equatorial continuity
set of spheres can be claimed either by the northern
or southern set of triangles and
squares, but they cannot serve both simultaneously.
Here again we have alternating
conditions__ starving or fulfilling__ of northern and southern
hemispheres matching or
nonmatching triangles and squares, with the central
equilibrium condition having a large
plurality of alternately realizable behaviors under
variously modified conditions affected
further as frequency increases the numbers of edge-vertex-occupying
spheres.
When modular frequency enters into the alternately
vector
equilibriumępolarized conformations, the vertexes of
the multifrequenced nuclear system
are occupied by uniradius spheres, whereat it is evidenced
that the equatorial continuity
set of spheres can be claimed either by the northern
or southern set of triangles and
squares, but they cannot serve both simultaneously.
Here again we have alternating
conditions__ starving or fulfilling__ of northern and southern
hemispheres matching or
nonmatching triangles and squares, with the central
equilibrium condition having a large
plurality of alternately realizable behaviors under
variously modified conditions affected
further as frequency increases the numbers of edge-vertex-occupying
spheres.
|

|
466.16
 As the frequencies of vector equilibria or icosahedra
increase, the relative
size of the occupied arcs of the great circles involved
become of ever lesser magnitude. At
a high frequency of larger spheres__ for example, planet
Earth__ the conditions of
patterning around the 12 external vertexes of the vector
equilibria or icosahedra appear to
be approximately flat, in contrast to the sharp concavity/convexity
of the nonfrequenced
convergence of the four planes around the corners of
the vector equilibrium and the
convergence of the five planes around the corners of
the icosahedron.
As the frequencies of vector equilibria or icosahedra
increase, the relative
size of the occupied arcs of the great circles involved
become of ever lesser magnitude. At
a high frequency of larger spheres__ for example, planet
Earth__ the conditions of
patterning around the 12 external vertexes of the vector
equilibria or icosahedra appear to
be approximately flat, in contrast to the sharp concavity/convexity
of the nonfrequenced
convergence of the four planes around the corners of
the vector equilibrium and the
convergence of the five planes around the corners of
the icosahedron.
|

|
466.17
 In very-high-frequency nuclear systems the approach
to flatness from the
four planes to five planes tends to induce a 360-degreeness
of the sums of the angles
around the critical 12 vertexes__ in contrast to the 300degree
condition existing in both the
unfrequenced vector equilibrium and icosahedron. That
is what Fig. 466.01 is all about.
In very-high-frequency nuclear systems the approach
to flatness from the
four planes to five planes tends to induce a 360-degreeness
of the sums of the angles
around the critical 12 vertexes__ in contrast to the 300degree
condition existing in both the
unfrequenced vector equilibrium and icosahedron. That
is what Fig. 466.01 is all about.
|

|
466.18
 In Figs. 466.01
and 466.41
there is introduced an
additional 60 degree
equilateral triangle, in surroundment of every directly-nuclear-emanating
vertex K. The 12
vector-equilibrium K vertexes are always in direct linear
relationship with the system
nucleus (see Sec. 414).
The additional degrees of angle
produced by the high-frequency
local flattening around K vertexes introduces a disturbance-full
exterior shell condition
that occasions energetic consequences of a centrifugal
character.
In Figs. 466.01
and 466.41
there is introduced an
additional 60 degree
equilateral triangle, in surroundment of every directly-nuclear-emanating
vertex K. The 12
vector-equilibrium K vertexes are always in direct linear
relationship with the system
nucleus (see Sec. 414).
The additional degrees of angle
produced by the high-frequency
local flattening around K vertexes introduces a disturbance-full
exterior shell condition
that occasions energetic consequences of a centrifugal
character.
|

|
466.20
 Centrifugal Forces
Centrifugal Forces
|

|
466.21
 As we get into ultra-ultra-high-frequency, and as
we get to greater and
greater sphericity, by virtue of the inherent spin,
we can account for the vector equilibrium
becoming the sphere of lesser radius, becoming the sphere
of approximately tetravolume
5, while the relative flatness around the critical K
vertexes relates to the centrifugal forces
involved.
As we get into ultra-ultra-high-frequency, and as
we get to greater and
greater sphericity, by virtue of the inherent spin,
we can account for the vector equilibrium
becoming the sphere of lesser radius, becoming the sphere
of approximately tetravolume
5, while the relative flatness around the critical K
vertexes relates to the centrifugal forces
involved.
|

|
466.22
 People think of centrifugal force as picturable by
arrows expelled radially
(perpendicularly) outward. But in fact centrifugal force
operates as a hammer-thrower's
hammer does: it departs from the system tangentially,
not radially. Since the outward
tangent ends reach ever farther away, there is a net
only-indirectly-radial force realized.
This common misapprehension of the assumed 180-degreeness
of centrifugal forces has
greatly misled human thinking and has obscured comprehensions
of precession.
People think of centrifugal force as picturable by
arrows expelled radially
(perpendicularly) outward. But in fact centrifugal force
operates as a hammer-thrower's
hammer does: it departs from the system tangentially,
not radially. Since the outward
tangent ends reach ever farther away, there is a net
only-indirectly-radial force realized.
This common misapprehension of the assumed 180-degreeness
of centrifugal forces has
greatly misled human thinking and has obscured comprehensions
of precession.
|

|
466.23
 At certain high frequencies the energy displacements
tend to occur that do
not tend to occur at low- or no-frequency conditions,
which brings us into the realm of
possibly comprehending the photon-emitting radiation
limits of operation within the 92
regenerative chemical elements and the split-second
articulatability of transuranium
nuclear systems when bombarded with ultraultra-high-frequency
energy missiles. The
lower the frequency, the higher the required bombardment
energies.
At certain high frequencies the energy displacements
tend to occur that do
not tend to occur at low- or no-frequency conditions,
which brings us into the realm of
possibly comprehending the photon-emitting radiation
limits of operation within the 92
regenerative chemical elements and the split-second
articulatability of transuranium
nuclear systems when bombarded with ultraultra-high-frequency
energy missiles. The
lower the frequency, the higher the required bombardment
energies.
|

|
466.30
 Nuclear Tetrahedra Pairs: Closest-sphere-packing Functions
Nuclear Tetrahedra Pairs: Closest-sphere-packing Functions
|
 Fig. 466.31 |
466.31
 In
Fig. 466.01-C
is a plan view of the closest-sphere-packing
manifestation
of any one of the vector equilibrium's four pairs of
nuclear tetrahedra as they commence
to torque in the jitterbug process. An isometric sketch
of this net 39-ball aggregation is
given in Fig. 466.31. Note that this torqued, north-south-pole,
axial pair of tetrahedra
employs three of the vector equilibrium's six axes.
The other three unengaged axes lying
in the equator are starved and inoperative__ angularly
acceleratable independently of the
north-south axial motion.
In
Fig. 466.01-C
is a plan view of the closest-sphere-packing
manifestation
of any one of the vector equilibrium's four pairs of
nuclear tetrahedra as they commence
to torque in the jitterbug process. An isometric sketch
of this net 39-ball aggregation is
given in Fig. 466.31. Note that this torqued, north-south-pole,
axial pair of tetrahedra
employs three of the vector equilibrium's six axes.
The other three unengaged axes lying
in the equator are starved and inoperative__ angularly
acceleratable independently of the
north-south axial motion.
|

|
466.32
 In
Fig. 466.01-C
we see the internal picture from
the nucleus to the vertexes
displaying the hexagonal pattern emerging at F3.
In
Fig. 466.01-C
we see the internal picture from
the nucleus to the vertexes
displaying the hexagonal pattern emerging at F3.
|

|
466.33
 There can be only one pair of tetrahedra operative
at any one time. The other
three pairs of tetrahedra function as standby auxiliaries,
as in the triangular-cammed, in-
out-and-around, rubber cam model described in Secs.
465.01
and 465.10.
There can be only one pair of tetrahedra operative
at any one time. The other
three pairs of tetrahedra function as standby auxiliaries,
as in the triangular-cammed, in-
out-and-around, rubber cam model described in Secs.
465.01
and 465.10.
|

|
466.34
 The active triangular face has to share its vertexes
with those of the adjacent
square-face grids. This transformation relates to the
transformation of the octahedron and
the rhombic dodecahedron.
The active triangular face has to share its vertexes
with those of the adjacent
square-face grids. This transformation relates to the
transformation of the octahedron and
the rhombic dodecahedron.
|

|
466.35
 In the outer layer of 92 balls__ two of which are extracted
for the axis of
spin__there are eight triangular faces. There are four
balls in the center of each of the six
square faces.
In the outer layer of 92 balls__ two of which are extracted
for the axis of
spin__there are eight triangular faces. There are four
balls in the center of each of the six
square faces.
|

|
6 × 4 = 24. 92 - 24 = 68. 68/8 = 8 l/2. |

|
We need 20 balls for a pair of complete polar triangles. |

|
68 - 20 = 48. 48/8 = 6; a pair of 6s = 12. Thus there are only 12 available where 20 are required for a polar pair. In any one hemisphere the vertex balls A, B, C used by a polar triangle make it impossible to form any additional polar units. |

|
466.40
 Universal Section of Compound Molecular Matrix
Universal Section of Compound Molecular Matrix
|

|
466.41
 The illustration at the back-end paper was first published
by the author in
1944. It displays the surface shell matrix of an ultra-high-frequency
sphere in which a local
planar flatness is approached. The vertexes are energy
centers, just as in the isotropic
vector matrix where 12 exterior corner vertexes of the
vector equilibria are always
connected in 180-degree tangential direct radial alignment
with the nuclear sphere.
The illustration at the back-end paper was first published
by the author in
1944. It displays the surface shell matrix of an ultra-high-frequency
sphere in which a local
planar flatness is approached. The vertexes are energy
centers, just as in the isotropic
vector matrix where 12 exterior corner vertexes of the
vector equilibria are always
connected in 180-degree tangential direct radial alignment
with the nuclear sphere.
|

|
466.42
 This compound molecular matrix grid provides a model
for molecular
compounding because it accommodates more than one tetrahedron.
This compound molecular matrix grid provides a model
for molecular
compounding because it accommodates more than one tetrahedron.
|

|
466.43
 This matrix is not isotropic. It is anisotropic. It
accommodates the domain of
a nucleus.
This matrix is not isotropic. It is anisotropic. It
accommodates the domain of
a nucleus.
|
| Next Section: 470.00 |